Beating Baccarat

In this post I am going to examine the earnings that are possible in baccarat if the player is using computer-perfect play. I investigated card counting in detail in this post. My method was standard: I determined the effect of removal for various cards, created card counting systems for the Banker bet and Player bet, and then simulated those counting systems.  The simulations demonstrated that card counting baccarat is a bust for the AP.

 

Card counting systems work on the theory that by keeping track of the relative density of groups of cards, the player can identify situations when the remainder of the shoe gives an edge over the house. In baccarat, card counting attempts to identify when either the Player bet or Banker bet is player-advantageous. But, in order for a card counting system to work in the first place, there must be actual situations when the player has the edge.

 

If an edge is going to occur, the situation will most likely occur late in the shoe. The strength of the opportunities will be proportional to the number of unseen cards.  In this study, I investigated situations where the number of unseen cards varied from 18 to 50 at the time of the wager. Beyond 50 cards, there were effectively no instances of a player edge over the house on either the Player or Banker wager. Likewise, most casinos use dealing procedures that do not afford players an opportunity to make a wager with fewer than 18 unseen cards left in the shoe.

 

Here is a summary of how I proceeded:

1. Fix a number of unseen cards. I ran simulations for 18, 26, 34, 42 and 50 unseen cards.
2. Shuffle the eight-deck shoe.
3. Deal out the shoe until the appropriate number of unseen cards is left.
4. Determine the exact edge of the remaining shoe by combinatorial analysis.
5. Record the exact edge in a log file.
6. Go to step 2.

 

After repeating steps 2 through 6 for one hundred thousand (100,000) baccarat shoes, I imported the information into Excel. I then plotted the distribution of the house edge for both the Player and Banker bets.  I did this for 18, 26, 34, 42 and 50 unseen cards, so altogether I ran five huge simulations.

 

It is worthwhile to look at one distribution in detail to get some intuition for the information I obtained. For example, here is the distribution for 18 unseen cards:

 

 

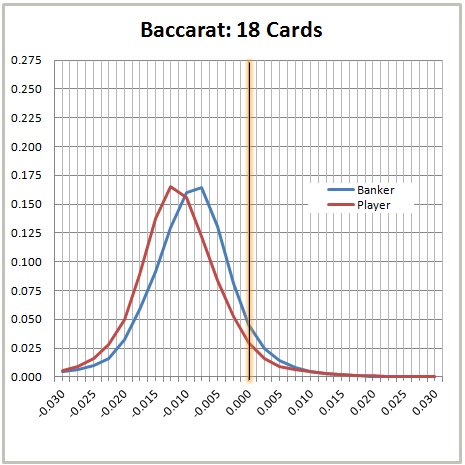
To read this graph, the horizontal axis gives the house edge. A negative number represents the house having the edge. A positive value represents the player having the edge: the portion to the right of the orange vertical line corresponds to the player having the edge over the house.  The tick-marks along the vertical axis correspond to the frequency with which a particular edge occurs. Going up the vertical axis corresponds to outcomes that are more likely.

 

Note that the red Player curve reaches a maximum value at -0.0124 (-1.24%), corresponding to the house edge for the Player bet. Likewise, the blue Banker curve reaches a maximum at -0.0106 (-1.06%), corresponding to the house edge for the Banker bet.

 

There are two geometric features about these curves I want to point out. These correspond to the "average edge" when the Banker/Player bet is favorable and the frequency of the opportunity to make an advantageous Banker/Player bet. These are the two numbers that allow the total potential income to be computed.

 

First, if you consider the portion under each curve to the right of zero, then the “center of gravity” of each curve corresponds to the average edge.  The area under the blue curve to the right of zero has “center of gravity” at 0.00679.  This corresponds to an average edge over the house of 0.679% if a Banker wager is made every time it is player-favorable with 18 unseen cards. The area under the red curve in the box has “center of gravity” at 0.00479. This corresponds to an average edge over the house of 0.479% if a Player wager is made every time it is player-favorable with 18 unseen cards.

 

Second, the total area under each curve in its entirety is exactly 1. The fraction under the curve to the right of zero corresponds to the frequency of a player-favorable wager. The area under the blue curve to the right of zero is 0.04589. It follows that a player-favorable wager on the Banker bet occurs 4.589% of the time with 18 unseen cards. The area under the red curve to the right of zero is 0.05909. It follows that a player-favorable wager on the Player bet occurs 5.909% of the time with 18 unseen cards.

 

In summary, with 18 unseen cards:

 

• The Banker bet will be player-favorable on 4.589% of the hands. When the player has an edge making the Banker bet, his average edge is 0.679%.
• The Player bet will be player-favorable on 5.909% of the hands. When the player has an edge making the Player bet, his average edge is 0.479%. 

The following tables summarize the pertinent data for the distributions above:

 

 

 

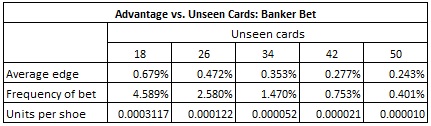
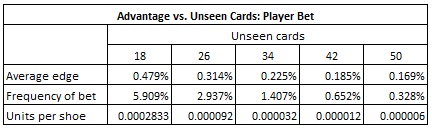
After each round is dealt from the shoe, the player will have an opportunity to make his next baccarat wager with 4-to-6 fewer unseen cards than the previous round. Using the data above, I was able to plot the total possible units that could be won per shoe using computer perfect play, based on the number of unseen cards at the start of the final round of the shoe. The following chart gives this information:

 

 

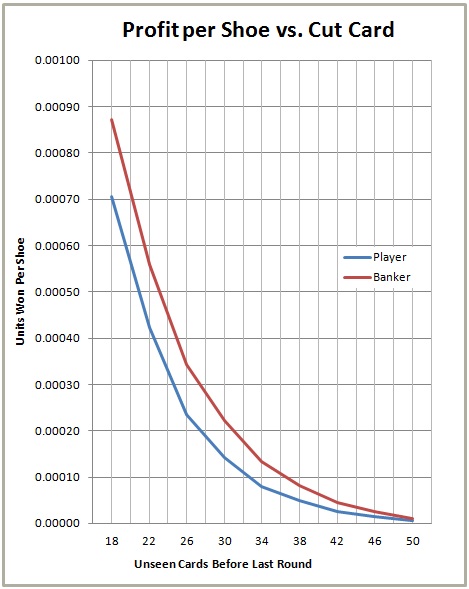
In particular, with the final round occurring with 18 unseen cards and with computer perfect play:

• The profit per shoe for the Player bet is at most 0.000705 units.
• The profit per shoe for the Banker bet is at most 0.000871 units.

 

No card counting system can exceed these practical limits. To put this in dollars and cents, suppose that an AP is using a card counting system to try to beat baccarat. This hypothetical player never wagers unless he has the edge over the house. When he has the edge on the Banker bet, he wagers $1000 on it. Whenever he has the edge on the Player bet, he wagers $1000 on it. Then:

• The profit per shoe for the Player bet is at most 70.5 cents per shoe.
• The profit per shoe for the Banker bet is at most 87.1 cents per shoe.
• The profit per shoe for making both Player and Banker bets is at most $1.58 per shoe.

 

Maybe he’s the world’s most perfect card counter. Or perhaps he is using a computer. Or maybe he is just psychic. It doesn’t matter. Just assume this player somehow knows exactly when he has the edge over the house and whenever he does, he bets $1000. The practical limitations on the earning potential for this player are profound -- $1.58 per shoe for a wager size of $1000.

 

In other words, baccarat cannot realistically be beaten by computer perfect play.  Occam's razor then dictates that a suspected advantage player is either lucky, using a stronger method (card sorts, ribbon spread, etc.), or is a crook.