Card Counting the Colour Match Baccarat Side Bet

While looking around for what to do next, I decided to visit one of my favorite sources for interesting baccarat side bets: the website ukcasinotablegames.info . There I found the “Colour Match” (CM) baccarat side bet. The CM side bet is based on the first four cards dealt in a round of baccarat, the two Player cards and the two Banker cards. If these four cards are all the same color (four red cards or four black cards), then the player is paid 6-to-1 for his CM wager, otherwise he loses. This side bet immediately struck me as easy to count using a simple red/black count.

 

The combinatorial analysis of CM is trivial. Here are the details for an eight-deck shoe. It shows a house edge of 13.7635%.

 

 

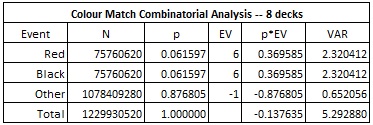
The following spread sheet can be used to compute the house edge for any shoe composition: Baccarat_Colour_Match

 

The easiest way to beat CM is to use a spread sheet like the one above, or a customized smart phone app, to compute the house edge in real-time as the cards are dealt. This vulnerability is similar to that of the Pairs bet (see this post) and is legal in certain jurisdictions worldwide. There is no reason to assume that players who are “playing by phone” are not using such a spread sheet.

 

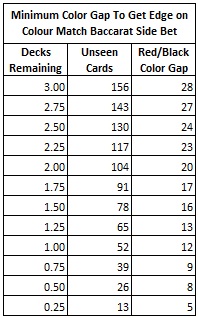
The AP gets an edge whenever there is a sufficiently large spread (color gap) between the number of red cards and black cards that remain in the shoe. For example, at the 2-deck point (104 unseen cards), the gap is 20. If there are at least 20 more red cards than black cards remaining in the shoe, then the AP has the edge. Conversely, if there are at least 20 more black cards than red cards, then the AP has the edge.

 

The following spread sheet contains the color gap for every number of unseen cards left in the shoe from 357 cards (color gap = 59) to 10 cards. There is no edge possible with more than 357 unseen cards remaining in the shoe. Colour_Bet_Minimum_Gap

 

The following table gives a sample of the color gaps, starting at the 3-deck point (156 unseen cards), by quarter-decks:

 

 

It is intuitively obvious that the best card counting system to use against CM is the red/black count. That is, red = +1, black = -1. There are then two trigger true counts, a positive one and a negative one. When the true count is sufficiently positive, then a winning CM wager using black cards is more likely. When the true count is sufficiently negative, then a winning CM wager using red cards is more likely.

 

Intuitively, I expected CM to be crushable using the red/black count. I was surprised to find that this is not the case. It appears that the relative safety of CM comes from the high off-the-top house edge.

 

Here are the results of a simulation of one billion (1,000,000,000) shoes of card counting CM using the red/black count:

 

 

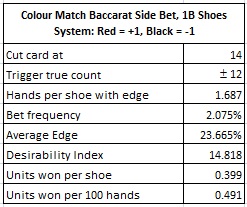
These numbers are similar to those for the Pairs bet (see this post), but CM is much easier to beat. Its DI (desirability index) is quite high, indicating that CM is an opportunity that would attract advantage play given sufficiently high table limits. This post discusses the DI of most of the baccarat side bets I previously analyzed. In particular, the only baccarat side bets with a higher DI are the natural 9/8 bets (natural 9/8 pays 8-to-1).

 

With a $100 table limit, the counter would earn about $40 per shoe, which is not enough to attract professional advantage play at the $100 level. I have visited several casinos that allow wagers over $10,000 on the Pairs bet. With similar table limits on CM, it becomes a significant game protection problem.

 

One number stuck out for me from the results of the simulation: the “average edge.” When the counter plays against the CM wager, his average edge is about 23.7%. This is an extraordinarily high value for the average edge. Towards the end of the shoe, even a difference of one red or black card can swing the edge through 10% or more.

 

The following graph gives the house edge vs. the true count, using the red/black count:

 

 

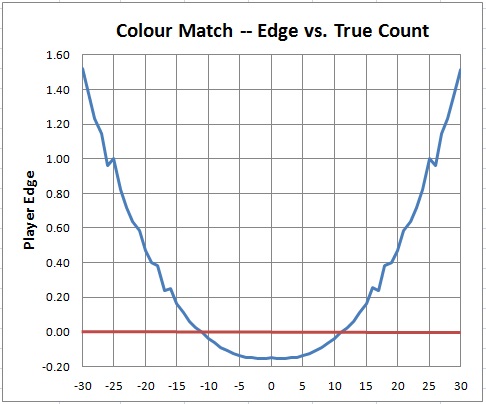
The edge quickly moves towards the player side when the true count moves beyond the trigger counts of ±12. The AP gets an edge of 20% over the house with a true count of ±15. At a true count of ±20, the counter's edge is over 40%. At a true count of ±25, the counter has about a 100% edge over the house! The data I collected (but did not graph) gives edges up to 300% at sufficiently high counts. It is conceivable that the last hand of the shoe comes from a shoe that only contains cards of the same color, at which point a win is guaranteed.

 

Although the overall win-rate against CM is modest, I view CM as highly vulnerable to advantage play. The ease of the card counting system together with the availability of high table limits gives the potential for significant profit. Casinos that offer CM together with deep cut-card placement should be aware of this possibility and put appropriate safeguards in place.